41. Bending moment at any section in a conjugate beam gives in the actual beam
deflection
42. For a two-hinged arch, if one of the supports settles down vertically, then the
horizontal thrust remains unchanged
43. For a symmetrical two hinged parabolic arch, if one of the supports settles horizontally, then the horizontal thrust is decreased
44. A single rolling load of 8 kN rolls along a girder of 15 m span. The absolute maxi
mum bending moment will be 30 kN m
45. The maximum bending moment due to a train of wheel loads on a simply supported girder always occurs under a wheel load
46.When a uniformly distributed load, longer than the span of the girder, moves from left to right, then the maximum bending moment at mid section of span occurs when the uniformly distributed load occupies whole span
47.When a uniformly distributed load, shorter than the span of the girder, moves from left to right, then the conditions for maximum bending moment at a section is that the load position should be such that the section divides the load in the
same ratio as it divides the span
48.When a series of wheel loads crosses a simply supported girder, the maximum
bending moment under any given wheel load occurs when the centre of span is midway between the centre of gravity of the load system and the wheel load under consideration
49.Which of the following is not the displacement method ?
Column analogy method
50. The Muller-Breslau principle can be used to determine the shape of the influence line, indicate the parts of the structure to be loaded to obtain the maximum effect,calculate the ordinates of the influence lines
51. The correct shear force diagram for the beam shown below is

52. The correct bending moment diagram for the middle column of the frame shown below is

53. The reaction at support A of the propped cantilever beam shown in Fig. 12.7 is
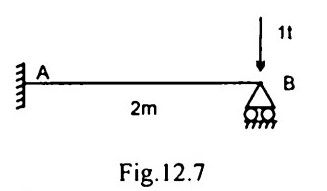
0
54

For unyielding supports, the slope deflection equation for part BC of the beam
shown in Fig. 12.8 is
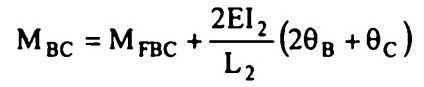
55.What is the degree of static indeterminacy of the structure shown in Fig. 12.9 ?
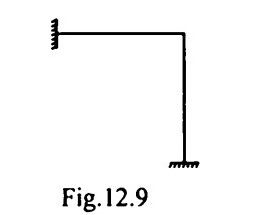
3
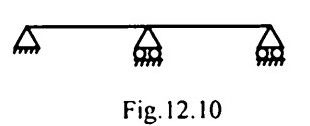
56.What is the degree of kinematic indeterminacy of the beam shown in Fig. 12.10?
5
57.What is the degree of kinematic indeterminacy of the beam shown in Fig. 12.10,
if the axial deformation is ignored? 3
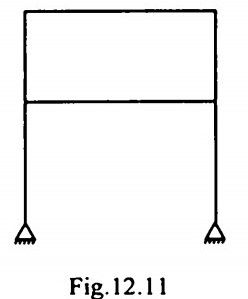
58.What is the degree of kinematic indeterminacy of the frame shown in Fig. 12.11,
if the axial deformation is ignored ? 8
59.What is the degree of static indeterminacy of the beam shown in Fig. 12.12 ? 2


60.The portal frame shown in Fig. 12.13 will sway towards right



Comentarios